Factorisation is the opposite process of expanding brackets For example, expanding brackets would require \ (2 (x 1)\) to be written as \ (2x 2\) Factorisation would be to start with \ (2x 2\) and end up with \ (2 (x 1)\) The two expressions \ (2 (x 1)\) and \ (2x2\) are equivalent; Factorise a 12 x 4 a 4 x 12 Share with your friends Share 7 a 12 x 4 – a 4 x 12 = a 4 x 4 (a 8We have to factorise x 4y 4 Solution x4 – y 4 cxn ye expressed xs (x 2) 2 – (y2) 2 —(i) We know the identity \(x^{2}y^{2}= (xy)(xy)\) Hence using the

How To Factorize X 1 X 2 X 3 X 4 15 Quora
X^4-16y^4 factorise
X^4-16y^4 factorise-Factorise x^24x4 Updated On 9 To keep watching this video solution for FREE, Download our App Join the 2 Crores Student community now! Factorise x4 x2 1 Factorise x4 x2 1 how_to_reg Follow thumb_up Like (11) visibility Views (714K) edit Answer question_answer Answers(2) edit Answer person Kishore Kumar Consider x 4 x 2 1 = (x 4 2x 2 1) – x 2 = (x 2) 2 2x 2 1 – x 2 = x 2 1 2 – x 2 It is in the form of (a 2 – b 2) = (a b)(a




Factorise X 4 4 Youtube
Algebraic Methods wwwnaikermathscom 5 f(x) = 3x3 – 5x2 – 16x 12 (a) Find the remainder when f(x) is divided by (x – 2)(2) Given that (x 2) is a factor of f(x), (a) factorise f(x) completely(4) June 07 Q2 6 f(x) = 2x3 – 3x2 – 39x (a) Use the factor theorem to show that (x 4) is a factor of f (x)(2) (b) Factorise f (x) completely I am trying to factor x 4 1 in to two multiplied polynomials Homework Equations My teacher gave us this hint that its factored form is (ax2bxc)(ax2bxc) The Attempt at a Solution First i assumed that a and c were equal to 1 so that when x 2 is multiplied by the other x 2 is gives me x 4 and 1 times 1 gives me 1This is the same as x 2 0x 4, so you need to find factors of 4 that add up to 0
This is similar to other factorisation problems such as x 2 5x 6 In this problem, you would find prime factors of 6 that add up to 5 (In this case 3, 2) Now, do the exact same with this problem!Factorisation Factorisation class 9 Factorise x^8y^8 Factorise x^4y^4 Factorise x^4y^4 Factorise x^8y^8 Factorise x^2y^2#factorisationofpolynomiaQuotient of x^38x^217x6 with x3;
Add to your resource collection Ref R19 Printable/supporting materials Fullscreen mode Teacher notes Question Solution Question Show that (x2 2y2)2 −4x2y2 =x4 4y4, ( x 2 2 y 2) 2 − 4 x 2 y 2 = x 4 4 y 4, and hence factorise x4 4y4 x 4 4 y 4Factor x^41 x4 − 1 x 4 1 Rewrite x4 x 4 as (x2)2 ( x 2) 2 (x2)2 −1 ( x 2) 2 1 Rewrite 1 1 as 12 1 2 (x2)2 −12 ( x 2) 2 1 2 Since both terms are perfect squares, factor using the difference of squares formula, a2 −b2 = (ab)(a−b) a 2 b 2 = ( a b) ( a b) where a = x2 a = x 2 and b = 1 b = 1 (x2 1)(x2 −1) ( x 2View more examples » Access instant learning tools Get immediate feedback and guidance with stepbystep solutions and Wolfram




3 Ways To Factor Algebraic Equations Wikihow



How To Factor Polynomials Easily
Factorise `(a) x^(4)4x^(2)16 " " (ii) x^(4)4`X 4 − (y z) 4 (x 2) 2 − ((y z) 1) 2 (x 2 − (y z) 2) (x 2 (y x) 2)X^4 324 (x^2)^2 18^2 That would be a perfect square if the term that is twice the product of the square roots of those two terms were added That's 2·18·x^2 or 36x^2 So we add that between those terms and then subtract it That's the same as adding 0 (x^2)^2 36x^2 18^2 36x^2 Factor the first three terms as (x^2 18) (x^2 18) or




Ex 2 4 Q24c 𝑥3 𝑥2 4𝑥 4 Factorise




Factorise X 4y 4 Xy Maths Questions
They have the same value for all values of \ (x 3 Let s = x y 2 so x, s, y make an arithmetic progression with difference name it d So x = s − d and y = s d Now E = ( s − d) 4 ( s d) 4 16 s 4 = 2 s 4 12 s 2 d 2 2 d 4 16 s 4 = 18 s 4 12 s 2 d 2 2 d 4 = 2 ( 9 s 4 6 s 2 d 2 d 4) = 2 ( 3 s 2 d 2) 2 Share answered May 1 ' at 1549 Answer3x^2 4x 3x 4x ( 3x 4 ) 1 ( 3x 4 )( 3x 4 ) ( x 1 )hope it helps




X 3 X 2 4x 4 Factorise Edurev Class 9 Question
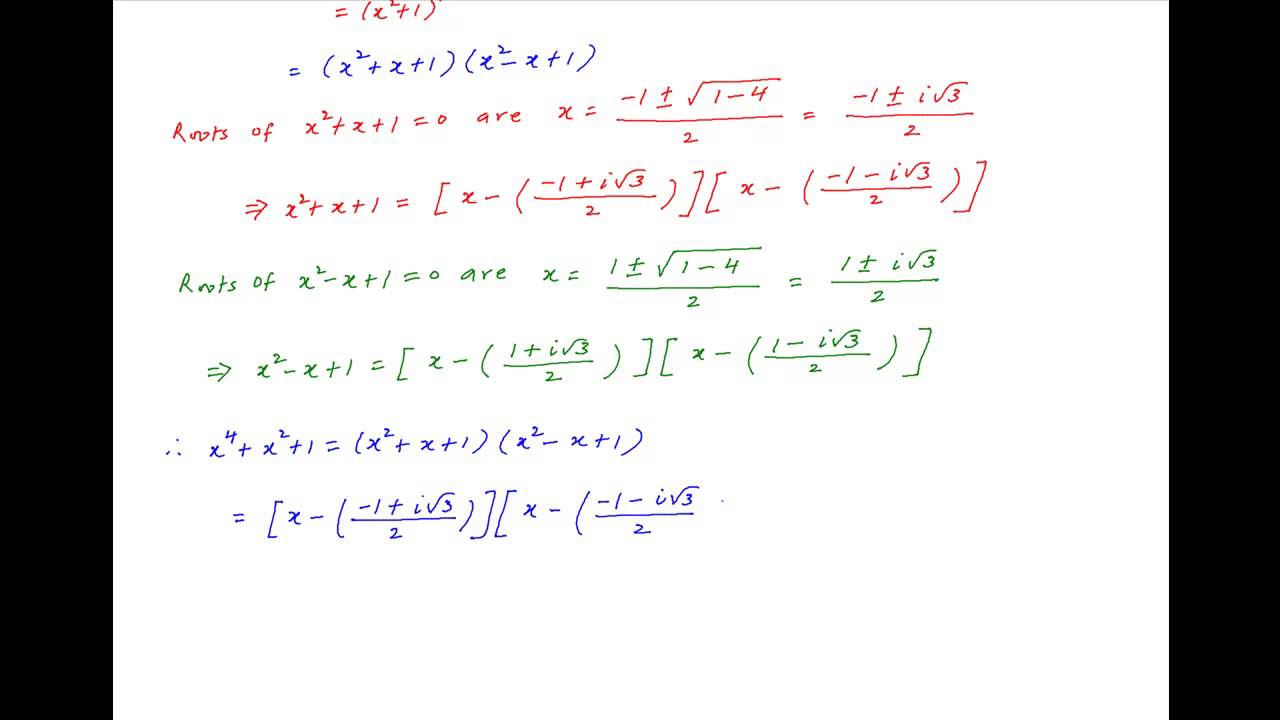



Factorize X 4 X 2 1 Into Linear Polynomials Youtube
Factorise 25/4 x^2y^2/9 Updated On To keep watching this video solution for FREE, Download our App Join the 2 Crores Student community now! Yes, the four solutions to x^44=0 are x=±1±i So to factor this completely, you have x^44=(x1i)(x1i)(x1i)(x1i) If you multiply the first two factors and the second two factors, you obtain x^44=(x^22x2)(x^2–2x2) Alternatively, if you multiply the first and last factor, and also the second and third, you get x^44=(x^2–2iWhich is what we wanted and hence factorise x4 4y4 x 4 4 y 4 We know that x4 4y4 =(x2 2y2)2 −4x2y2 x 4 4 y 4 = ( x 2 2 y 2) 2 − 4 x 2 y 2 Notice that the right hand side is of the form a2 −b2 a 2 − b 2, where a = x2 2y2 a = x 2 2 y 2 and b =2xy b = 2 x y



How To Factorise X X 1 Quora



Factorisation Definition Formulas And Factors Of Quadratic Equations
Factorise x 4 /4 4/x 4 1 Share with your friends Share 3 x 4 4 4 x 4Try typing these expressions into the calculator, click the blue arrow, and select "Factor" to see a demonstration Or, use these as a template to create and solve your own problems Problem 4x2 −9 4 x 2 − 9 Solution (2x3)(2x −3) ( 2 x 3) ( 2 x − 3) Problem x4 −81 x 4 − 81Factoriser Factoriser Enter a quadratic equation to factorise For indices use (^) Factorise Submit




Factorise X4 4 4 X 4 1



Using Factor Theorem Factorise The Polynomial X3 X2 4x 4 Studyrankersonline
Answer to Factorise \\text{x}^{4} \\text5{x}^{2} 6 By signing up, you'll get thousands of stepbystep solutions to your homework questions YouFor example putting 2x² x 3 into the form (2x 3)(x 1) factorise 9x to the power of 4 64y to the power of 2 Math Factorise X'29X 14 maths factorise a^7ab^6 You can view more similar questions or ask a new question x^4(xz)^4 can be factorised as, =x^4(xz)^4 =(x^2)^2(xz)^2^2 =x^2(xz)^2x^2(xz)^2 we used the following formula, a^2b^2=(ab)(ab) x(xz)x(xz)x^2x^2z^22xz we used the following formula, a^2b^2=(ab)(ab) (ab)^2=a^2b^22ab =x(xz)x(xz)x^2x^2z^22xz =(xxz)(2xz)2x^2z^22xz =z(2xz)2x^2z^22xz




Factoring Calculator Wolfram Alpha




How To Factorize X 1 X 2 X 3 X 4 15 Quora
Factorise x^4x^21 Get the answer to this question and access a vast question bank that is tailored for studentsExpand polynomial (x3)(x^35x2) GCD of x^42x^39x^246x16 with x^48x^325x^246x16; Ex 142, 4 Factorise (iv) 𝑥^4 – 〖"(x – z)" 〗^4 𝑥^4 – 〖"(x – z)" 〗^4 = 〖(𝑥^2)〗^2 − (𝑥 − z)^2 ^2 Using 𝑎^2 − 𝑏^2 = (a b) (a − b) Here 𝑎=𝑥^2 and b = (𝑥 − z)^2 = 𝑥^2(𝑥 − z)^2 𝑥^2 −(𝑥 − z)^2 Asking Using 𝑎^2 − 𝑏^2 = (a b) (a − b) Here 𝑎=𝑥 and b = (𝑥 − z) Asking Using 𝑎^2 − 𝑏^




Also A Factor And Deduce The Third Factor 7 Th Gauthmath




X4 X Z 4 Factorize Than Using Identity B2 Brainly In
Check x 4 is the square of x 2 Check y 4 is the square of y 2 Factorization is (x 2 y 2) • (x 2 y 2) Trying to factor as a Difference of Squares 12 Factoring x 2 y 2 Check x 2 is the square of x 1 Check y 2 is the square of y 1 Factorise Completelyx 4 – 2x 2 y 2 y 4 Answers x 4 – x 2 y 2 – x 2 y 2 y 4 x 2 (x 2 – y 2) – y 2 (x 2 – y 2) (x 2 – y 2) (x 2 – y 2) (x y) (x – y) (x y) (x – y) Kavungya answered the question on at 1045 Next Janice, a fruit vendor obtained a total of Kshs 6144 from her sales of Expression #=x^4y^4# Recall the factorization of the difference of two squares #a^2b^2 = (ab)(ab)# In our example, we will use this factorization twice Note #x^4 =(x^2)^2 and y^4 =(y^2)^2 # Applying the factorization above Expression #= (x^2y^2)(x^2y^2)# Now, the second factor above is also the difference of two squares




Online Factoriser With Steps



Www Pearson Com Content Dam One Dot Com One Dot Com Uk Documents Subjects Mathematics Worksheets Chapter2 Example 1 Chapter 2 Solving Quadratic Equations Pdf
Share It On Facebook Twitter Email 1 Answer 1 vote answered by Mohini01 (677k points) selected by Sunil01 Best answer x 4 – y 4 = (x 2) 2 – (y 2) 2 Identity a 2 – b 2 = (a b)(a – b)Here again we add and subtract suitable terms to bring it in a factorisation form Notice that x 4 4 y 4 = (x 2) 2 (2 y 2) 2 − 2 (x 2) (2 y 2) − (2 x y) 2 = (x 2 2 y 2) 2 − (2 x y) 2 The second expression is in the form a 2 − b 2, where a = (x 2 2 y 2) and b = 2 x y Hence we may write x 4 4 y 4 = (x 2 2 y 2 2 x y) (x 2 2 y 2 − 2 x y)Remainder of x^32x^25x7 divided by x3;




Rd Sharma Class 9 Chapter 5 Factorization Of Algebraic Expressions Updated For 21 22




Factorise X 4 1 X 4 1 Draw Cheerio
SolutionShow Solution `x^4 y^4 27x^2y^2` = ` (x^2)^2 (y^2)^2 2x^2y^2 25x^2y^2` = ` (x^2 y^2)^2 25x^2y^2` = ` ( x^2 y^2 )^2 (5xy)^2` ∵ a2 b2 = ( a b ) ( a b ) = ` (x^2 y^2 ) 5xy ( x^2 y^2 ) 5xy` = ` x^2 5xy y^2 x^2 5xy y^2 `Watch Video in App This browser does not support the video element 1603 k 1190 k Answer Step by stepSo x^4 7x^2 8 = y^27y8 y^27y8 = (y8) (y1) substitute x back in (x^2 8) (x^21) Now to simplify this, I don't think you can factorise the first bracket with whole numbers but you can simplify the second bracket (x^21) = (x1) (x1) so x^4 7x^2 8 = (x^2 8) (x1) (x




Why Is X 4 16 When Factored Not Just X 2 4 X 2 4 Brainly Com



Factorize X 4y 4 Xy Sarthaks Econnect Largest Online Education Community
how do you factorise?Example 1 with x coefficient and a constant Fully factorise x2 6x 5 x 2 6 x 5 Write out the factor pairs of the last number (5) in order x2 6x 5 x 2 6 x 5 Factors of 5 1, 5 2 We need a pair of factors that to give the middle number ( 6) and to give the last number ( 5 ) If you can't find rational roots with the Rational Root Theorem, your next bet for solving this on paper is to factor the quartic into two quadratic equations For example, in this case, we can let 64 x 4 64 x 3 − x 2 − 51 x 39 = ( a x 2 b x c) ( p x 2 q x r) Note that, by comparing coefficients, we can tell that a p = 64, c r = 39,




Factoring 4th Degree Polynomials
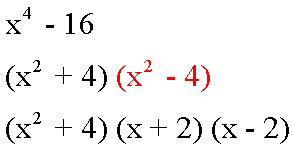



How To Factor 10 Steps With Pictures Instructables
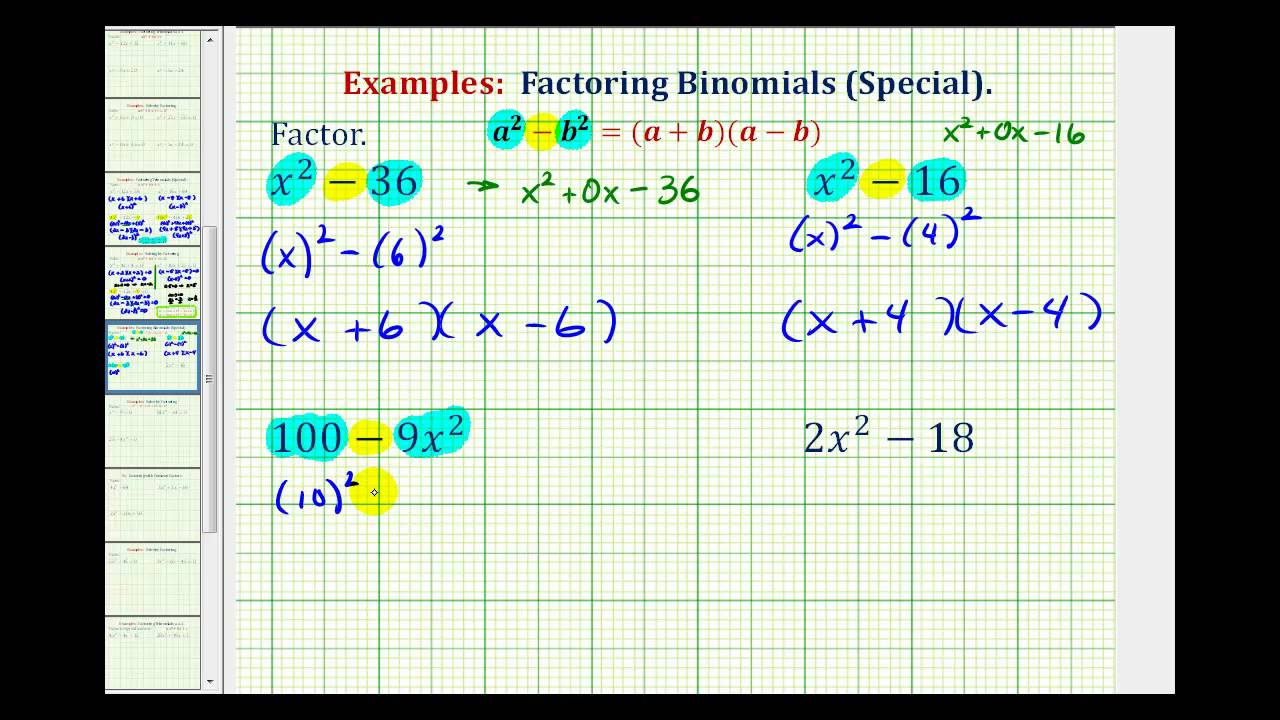
Transcript Ex 24, 4 Factorise 12x2 7x 1 12x2 7x 1 We factorize using the splitting the middle term method = 12x2 4x 3x 1 = 4x (3x 1) 1 (3x 1) = (3x 1) (4x 1) Ex 24, 4 Factorise (ii) 2x2 7x 3 2x2 7x 3 We factorize using the splitting the middle term method = 2x2 6x x 3 = 2x (x 3) 1 (x 3) = (x 3) (2x 1) Ex 24, 4 Factorise (iii) 6x2 5x 6 6x2 5x 6 WeFactor x^24 x2 − 4 x 2 4 Rewrite 4 4 as 22 2 2 x2 − 22 x 2 2 2 Since both terms are perfect squares, factor using the difference of squares formula, a2 −b2 = (ab)(a−b) a 2 b 2 = ( a b) ( a b) where a = x a = x and b = 2 b = 2 (x2)(x− 2) ( x 2) ( x 2)Factor x 4 324 (x 2) 2 18 2 That would be a perfect square if the term that is twice the product of the square roots of those two terms were added



Factoring Special Cases College Algebra




Factoring Using Polynomial Division Video Khan Academy
X 4 coefficient is 1 we know the coefficient for the x 2 terms in the factor will also be 1 (x 2) (x 2) Because the constant is a negative and the coefficient for the x term is a negative we know the sign for the constants in the factors will have one positive and one negative (x 2 ) (x 2 −) Now we need to determine the Factorise x 4 – y 4 factorisation;X 4 y 4 3x 2 y 2 = x 4 y 4 2x 2 y 2 x 2 y 2 = (x 2) 2 (y 2) 2 2x 2 y 2 x 2 y 2 = ( x 2 y 2) 2 (xy) 2 = ( x 2 y 2 xy )( x 2 y 2 xy ) ∵ a 2 b 2 = ( a b )( a b )
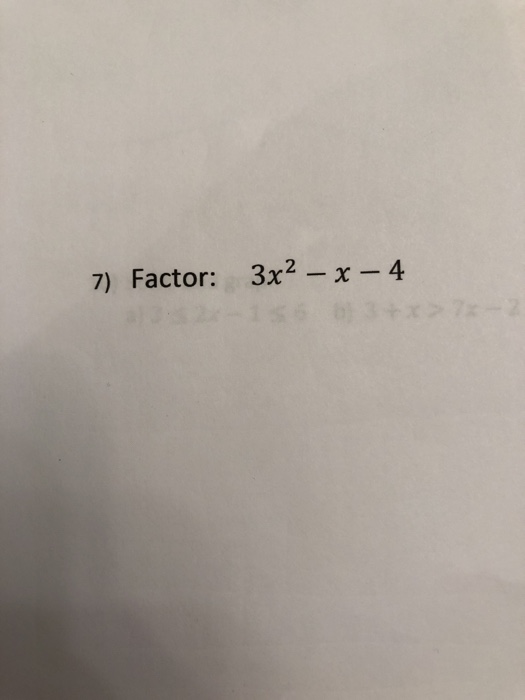



7 Factor 3x2 X 4 Chegg Com




Online Factoriser With Steps
Math\text{There are multiple ways to approach this problem}/math Approach # 1 mathx^4 x^2 1 = x^4 x^3 x^2 x^3 x^2 x x^2 x 1 /math mathQuestion what is the process to factorise x^4 324 Answer by Edwin McCravy() (Show Source) You can put this solution on YOUR website!Factorisation Zigya App Factorise x 4 (y z) 4 ∵ x 4 (y z) 4 = x 2 2 (y z) 2 2 = (x 2) (y z) 2 (x 2) (y z) 2 Using a 2 b 2 = (ab) (ab) We can factorise x 2 (y z) 2 further as x 2 (y z) 2 = (x) (y z) (x) (y z)
.png)



Factorise X4 4 X4 Maths Polynomials Meritnation Com




How To Factorise A Polynomial By Splitting The Middle Term A Plus Topper
Get answer Factorise (a) x^(4)4x^(2)16 " " (ii) x^(4)4 Apne doubts clear karein ab Whatsapp par bhi Try it nowWatch Video in App This browser does not support the video element 600 100 AnswerFactor x^45x^24 full pad » x^2 x^ {\msquare} \log_ {\msquare} \sqrt {\square} \nthroot \msquare {\square} \le \ge




Factoring X 4 Polynomials Completely Youtube
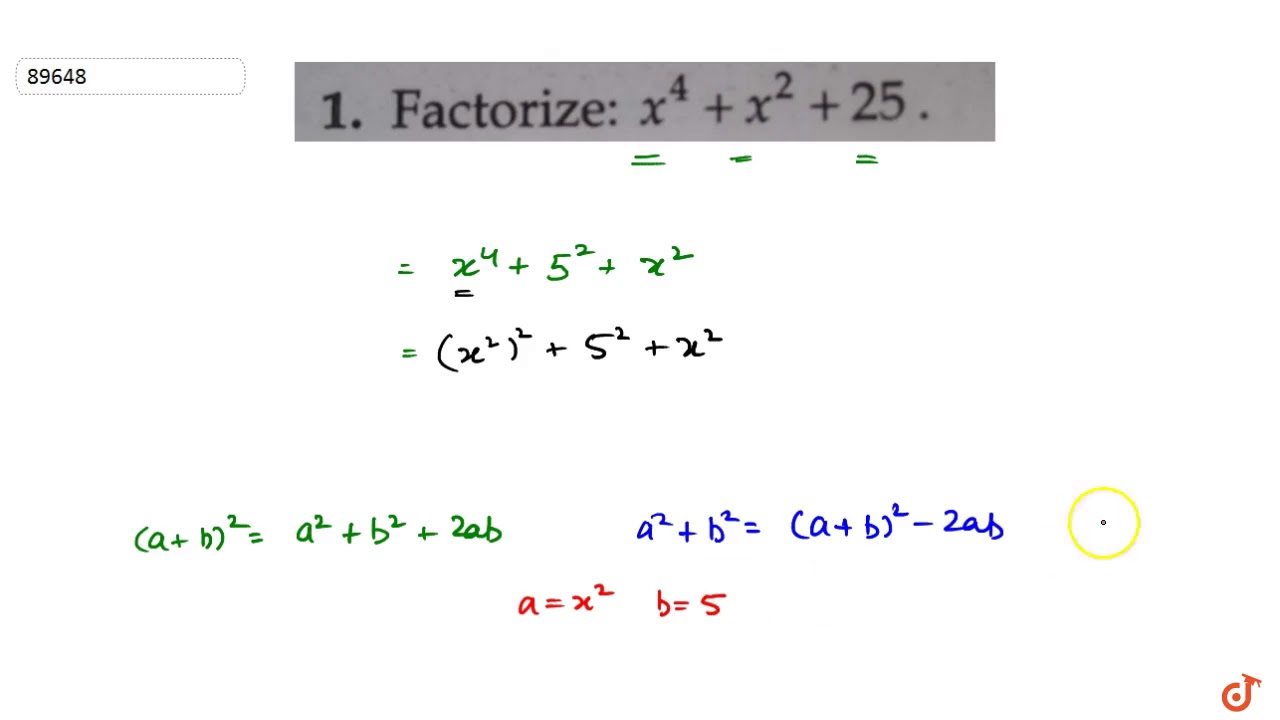



Factorise X 4 X 2 25 Youtube
Using factor theorem, factorise the polynomial x3 x2 4x 4 0 votes 137k views asked in Class IX Maths by priya12 (12,194 points) Using factor theorem, factorise the polynomial x 3 x 2 4x 4 polynomials




Factorize X 2 X 4 1 8 Youtube




Factorize X 4 4 Maths Factorization Of Algebraic Expressions Meritnation Com




Factorise X 4 4 4 X 4 1 By A 2 B 2 Brainly In




Ex 14 2 4 Iv Factorise X 4 X Z 4 Chapter 14 Class 8




1 1 2 Xx 2 2x X 1 2 Factorise The Following 1 Gauthmath



Factor X 4 1 Problem With Solution Lunlun Com




Factorise 3x 2 X 4 Maths Questions




X 4 14x 2 1 Factorize It Please Just Now Brainly In




Using The Factor Theorem Show That X 2 Is A Factor Of X 3 X 2 4 X 4 Hence Factorise The Polynomial Completely Mathematics Shaalaa Com
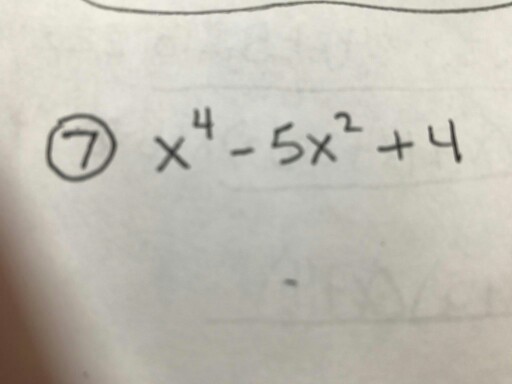



Factor X 4 5x 2 4 Chegg Com




Factorise X 1 X 2 X 3 X 4 3 Mathematics Topperlearning Com B0sdcrr



Factor X 2 4 Factor X Squared Plus 4




How To Factor Math X 4 4x 3 2x 2 4x 1 Math Quora




How To Factor Math X 4 15x 2 10x 24 Math Quora




3 Ways To Factor Trinomials Wikihow




Factorise X 4 X 2 1




Factorise X 2 3x 4




Factorise X 4 4 Youtube



1



1




X Power 4 Plus 4 Factorise Its Answer Is X Power 2 Plus 2x Plus 2 X Power 2 Minus 2x Plus 2 Brainly In




Factorize I X 4 Y 4 Ii 16 X 4 81 Iii X 4 Y Z 4 Iv 2x 32 X 5 V 3a 4 48 B 4 V Youtube



Factorise X4 X2 1 Polynomials Maths Class 9



Factorise X 2 3x 4




Solved X Square 4 Y Square 4 X 4 Factorise It Self Study 365
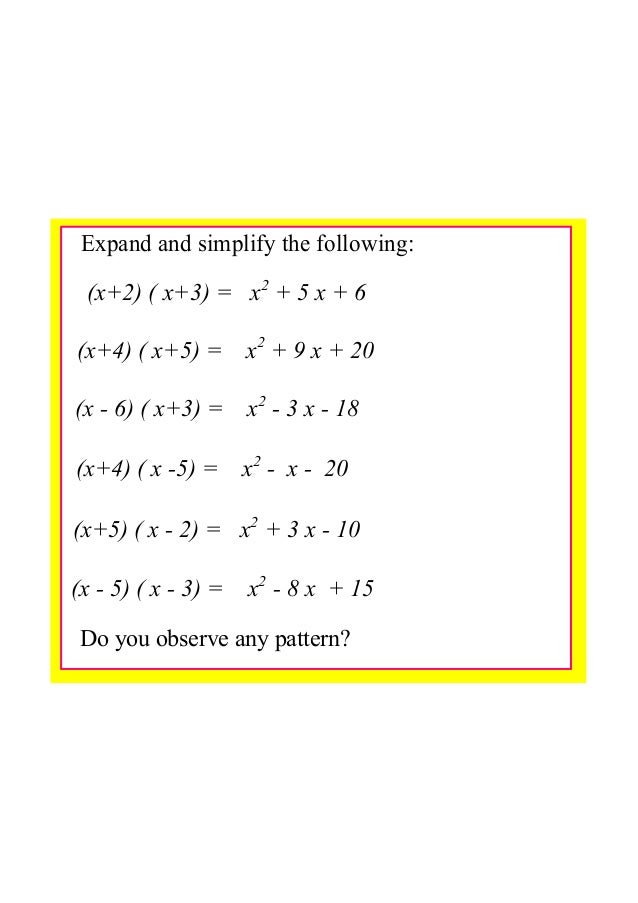



Factorising Quadratic Expressions 1
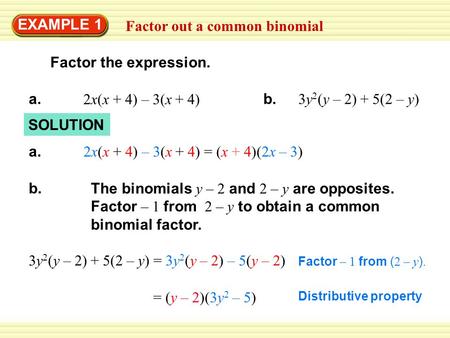



Unit 4 Review Solutions A 5 32a 2 4a 2 3a 3 8 2 A 2 B 2 Ab Ab Ab 1 3 X X 2 Y 2 X X X 2 Y X 2 X 2 X Y Ppt Download




Factoring Special Binomials




Factorise X2 X 4 1 8 Mathematics Topperlearning Com 057ly522




7 2 Factorisation Factorise Completely C 5x2y X 4 Gauthmath
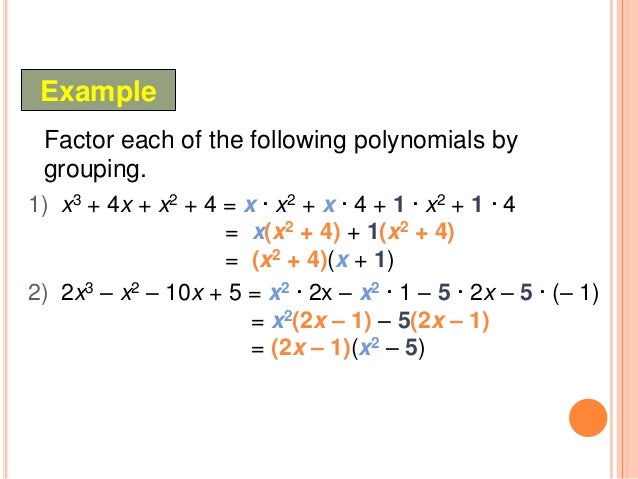



Factoring Polynomials




Ex 14 2 4 Iii Factorise X 4 Y Z 4 Chapter 14 Class 8




How To Factorise A Polynomial By Splitting The Middle Term A Plus Topper




X4 5x2 9 Factorize This Maths Factorisation Meritnation Com




Ex 14 2 4 Iv Factorise X 4 X Z 4 Chapter 14 Class 8



Www Pearson Com Content Dam One Dot Com One Dot Com International Schools Pdfs Secondary Curriculum International Old Maths Ial Pure Maths 2 Sb Pdf



How To Factorise X 3 3x 2 4 Quora




Factorise X 2 4x 4



Factorize X 3 12x X 4 64 Sarthaks Econnect Largest Online Education Community




X 4 4x 3 6x 2 4x 1 0 Mathematics Stack Exchange




Factorise A X 4 4x 2 16 Ii X 4 4
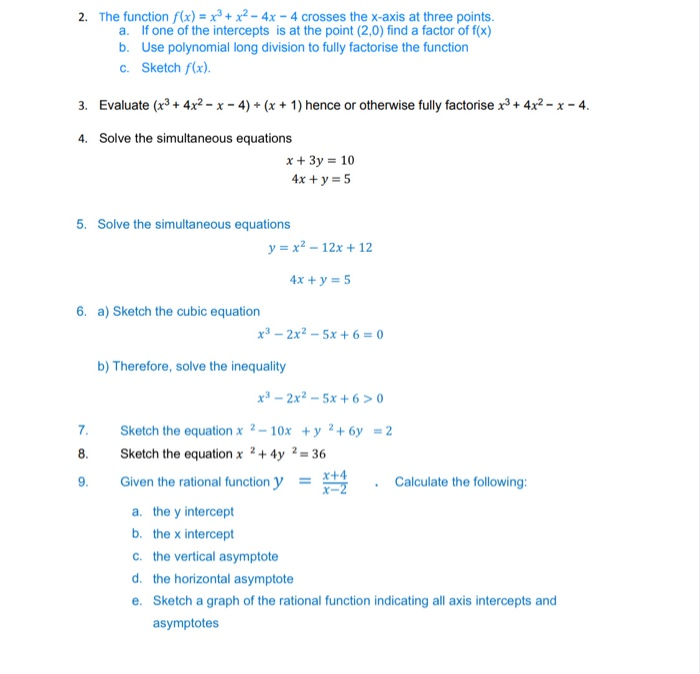



2 The Function F X X3 X2 4x 4 Crosses The Chegg Com



Can You Factor Math X 4 81 Math Quora




Factorise X 2 3x 4




Factorise X 4 X Z 4 Brainly In



How To Factorize X 4 X 2 1 Quora




Example 3 Factor By Grouping Factor The Polynomial X 3 3x 2 16x 48 Completely X 3 3x 2 16x 48 Factor By Grouping X 2 16 X 3 Distributive Ppt Download



View Question Factor X 4 3x 2 2
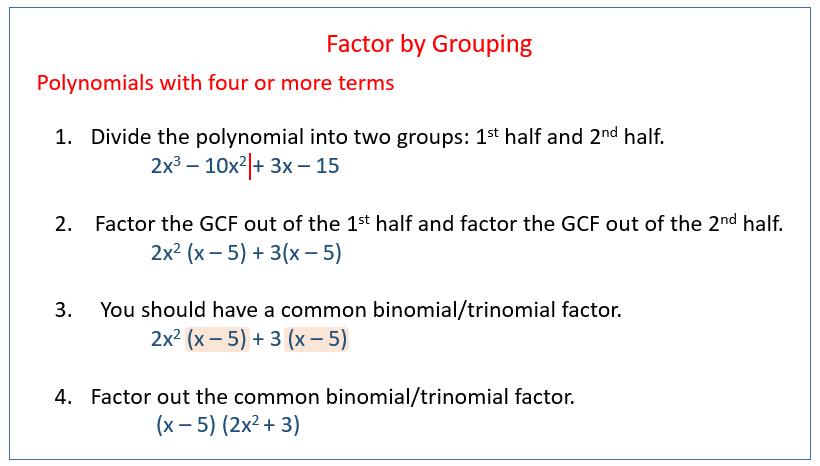



Factoring By Grouping Solutions Examples Videos




Ex 14 2 1 V Factorise 4x 2 8x 4 Chapter 14 Class 8




Using Factor Theorem Factorize The Polynomial X 4 2x 3 13x 2 14x 24




Factoring Polynomials




X 6 X 2 1 X2 8x X2 16x 48 X 12 X 4 Ppt Video Online Download




Factorise X Raised To Power 4 4 Maths Factorisation Meritnation Com




Factorise A X4 Y Z 4 Brainly In




Factor X 4 9 Youtube




Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes




How To Factor A Trinomial In 3 Easy Steps Mashup Math




Factorise X 4 1 Please Answer Brainly In




Using Factor Theorem Show That G X Is A Factor Of P X When P X X 4 X 2 12 G X X 2




Factors Learning Outcomes Ppt Download
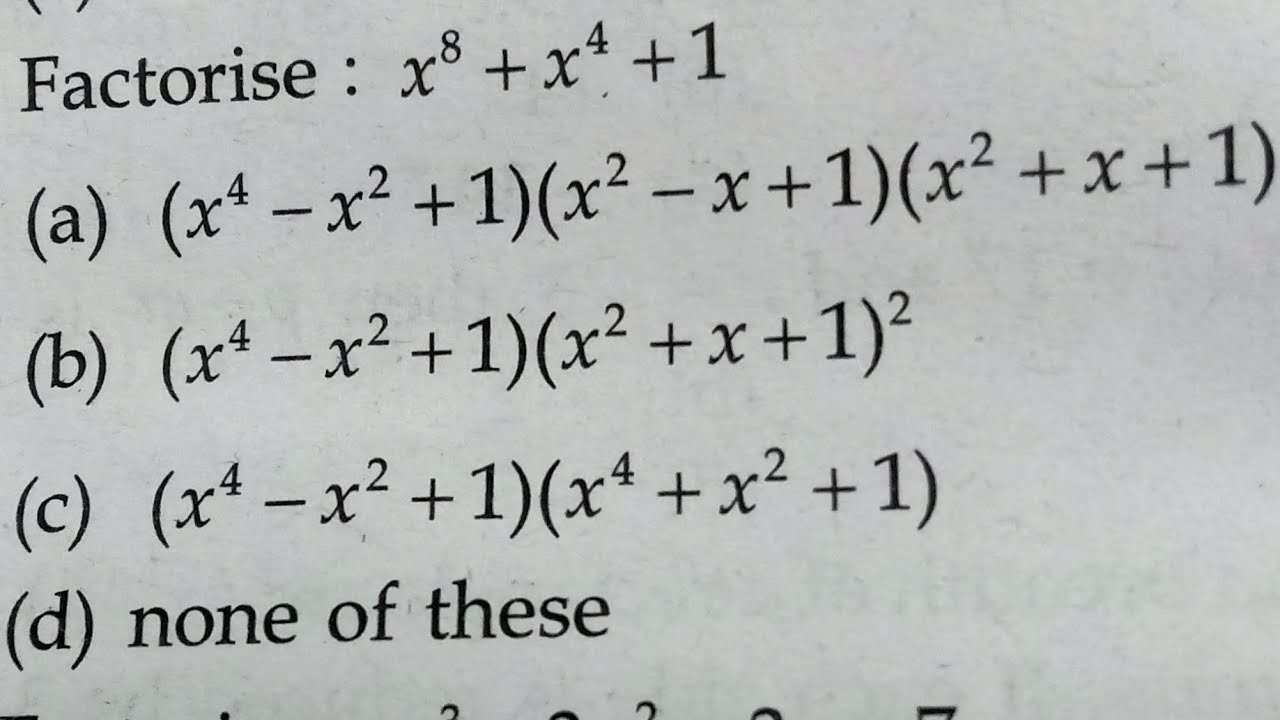



Factorise X 8 X 4 1 Youtube




Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes




What Is The Factor Of X Power 4 4 Maths Factorisation Meritnation Com



1




5 1 Expand And Simplify The Expression 3m N M 2mn Chegg Com




How To Factor Math X 4 X 3 7x 2 X 6 Math Quora
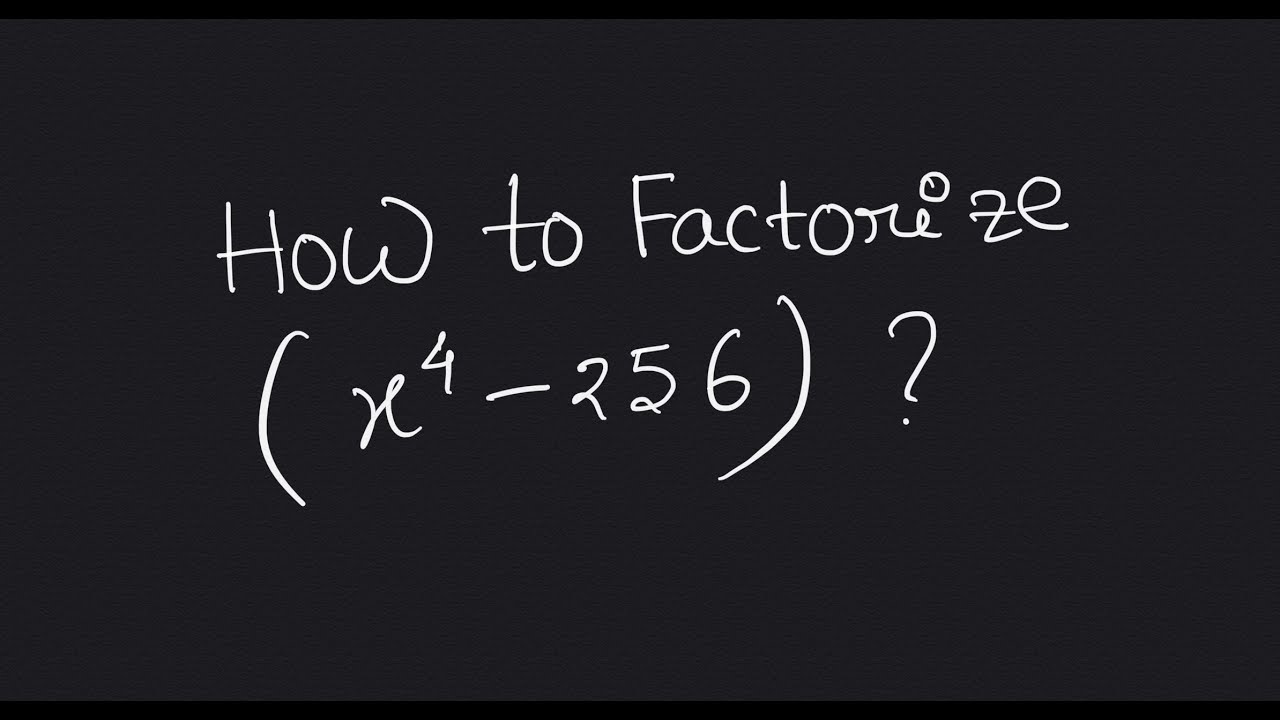



Factorize X 4 256 Youtube
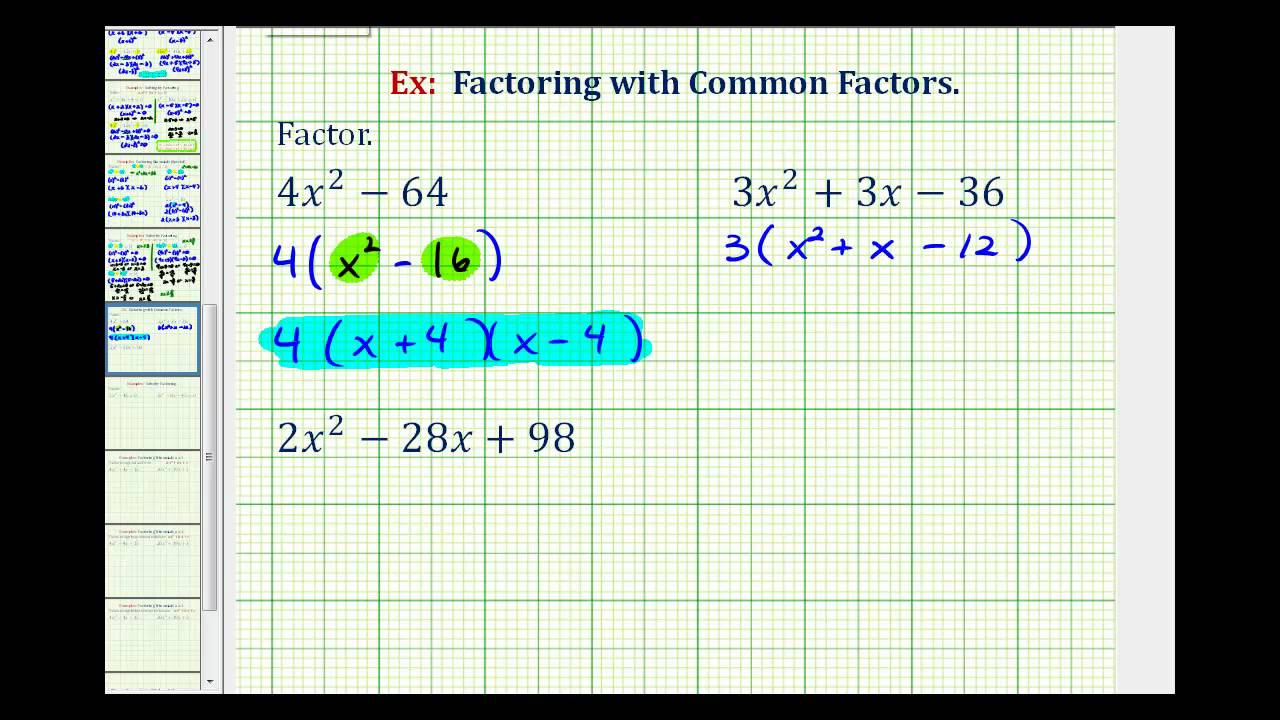



Factoring By Grouping Solutions Examples Videos
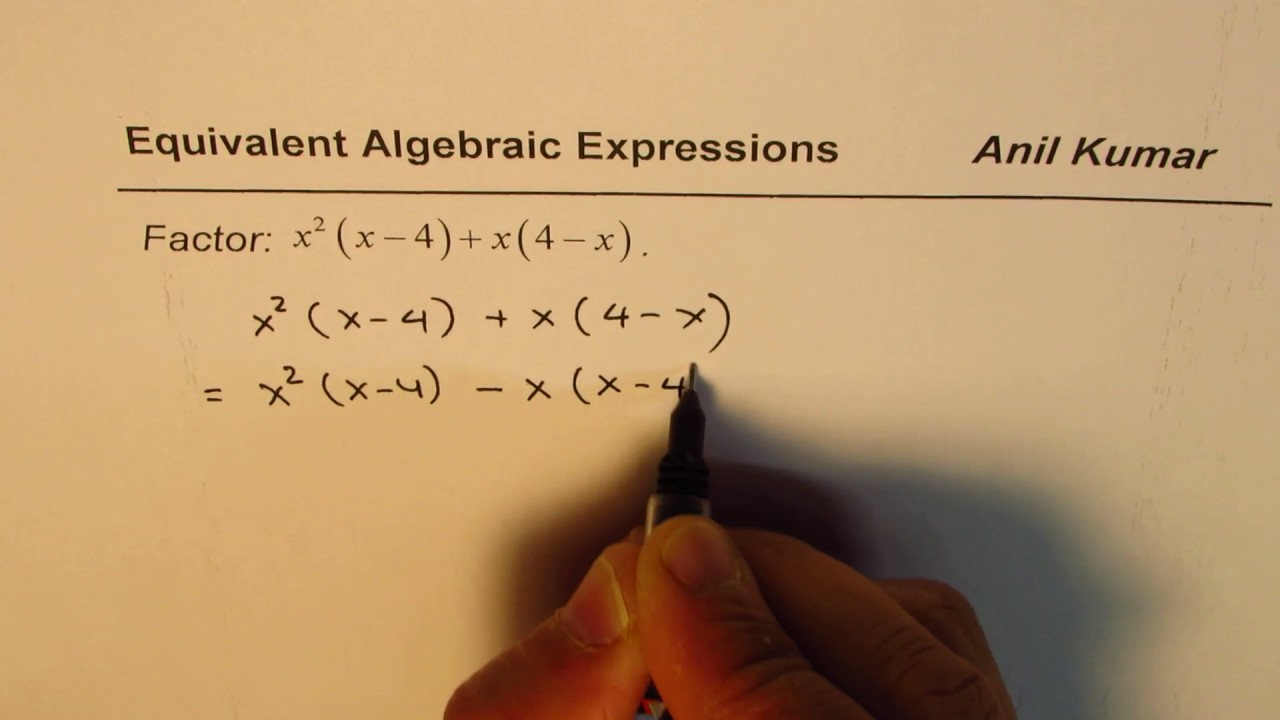



Factor Algebraic Expression X 2 X 4 X 4 X Youtube



Math Scene Equations Iii Lesson 2 Quadratic Equations



1
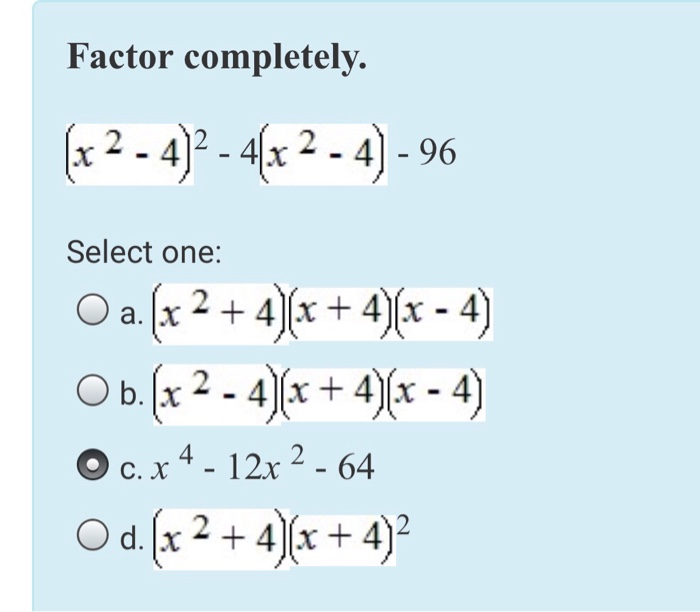



Factor Completely X 2 412 4x 2 41 96 Select One Chegg Com




Factorize X 4 16 Into Linear Polynomials Youtube



0 件のコメント:
コメントを投稿